 Gergő Almádi, member of our research group (to the left) won the Gyula Strommer Scholarship in 2024. The Scholarship is offered each year by the Gyula Strommer International Foundation of Geometry for one student of the BME. Congratulations!
Gergő Almádi, member of our research group (to the left) won the Gyula Strommer Scholarship in 2024. The Scholarship is offered each year by the Gyula Strommer International Foundation of Geometry for one student of the BME. Congratulations!
Successful PhD defense
Congratulations to Balázs Ludmány, who successfully defended his PhD thesis: An algorithmic approach to classical and mechanical shape descriptors of sedimentary particles (supervisor: Imre Szeberényi, Gábor Domokos).

New paper on optimal shapes of snail shells
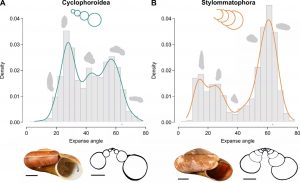
Many roads to success: alternative routes to building an economic shell in land snails
Barna Páll-Gergely, András Á. Sipos, Mathias Harzhauser, Aydın Örstan, Viola Winkler, Thomas A. Neubauer.
Abstract: Land snails exhibit an extraordinary variety of shell shapes. The way shells are constructed underlies biological and mechanical constraints that vary across gastropod clades. Here, we quantify shell geometry of the two largest groups, Stylommatophora and Cyclophoroidea, to assess the potential causes for variation in shell shape and its relative frequency. Based on micro-computed tomography scans, we estimate material efficiency through 2D and 3D generalizations of the isoperimetric ratio, quantifying the ratios between area and perimeter of whorl cross-sections (2D) and shell volume and surface (3D), respectively. We find that stylommatophorans optimize material usage through whorl overlap, which may have promoted the diversification of flat-shelled species. Cyclophoroids are bound to a circular cross-section because of their operculum; flat shells are comparatively rare. Both groups show similar solutions for tall shells, where local geometry has a smaller effect because of the double overlap between previous and current whorls. Our results suggest that material efficiency is a driving factor in the selection of shell geometry. Essentially, the evolutionary success of Stylommatophora likely roots in their higher flexibility to produce an economic shell.
Giovanni Barla Best Paper Award for Balázs Ludmány and coauthors
The paper A New Insight into the Stability of Precariously Balanced Rocks, authored by Balázs Ludmány, Gábor Domokos, András Sipos and others, was recognized by the Giovanni Barla Best Paper Award of 2023. The award is offered annually by the editors of Rock Mechanics and Rock Engineering to two or three papers published in the above journals. Congratulations!
Two lecturers in the top 100 of the BME
János Török and Krisztina Regős, members of our research group, are ranked 31st and 92th among all lecturers of the Budapest Univeristy of Technology and Economics.
Congratulations!
József Nádor Prize for Gergő Almádi
Gergő Almádi, student of the research group was awarded by József Nádor Prize on 12th March. The prize is traditionally given by the BME to students with outstanding candidature for the National Scholarship for Higher Education.
An interview with Krisztina Regős and Gergő Almádi at www.bme.hu
An interview with two young members of the HUN-REN Morphodynamics Research Group is published at www.bme.hu. Krisztina Regős and Gergő Almádi talk about the motivation of their research and experiences in publication.
Lectures for high school students
A series of scientific informative lectures for interested high school students is organized by the HUN-REN Morphodynamics Research Group.
Past events:
- Kocsis Kinga: Ezer tonna márvány, avagy tér-idő utazás Michelangelo kőtömbjeihez (A thousand ton of marble or a time-space travel to the stones of Michelangelo)
- Regős Krisztina: A diszkrét Gömböc nyomában (In the wake of a discrete Gömböc)
(Lovassy László High School, Veszprém, 8 December, 2023)
- Almádi Gergő: Tetraéderek egyensúlyairól (On equilibria of tetrahedra)
- Nagy Klaudia: Falak geometriája (The geometry of walls)
(Deák Ferenc High School, Budapest, 23 January, 2024) Link
Upcoming events:
(Árpád High School, Budapest, 27 March, 2024)
(Teleki Blanka High School, Budapest, 23 April, 2024)
A chapter in Hungarian Space Caleidoscope 2023/24
A chapter is dedicated to the HUN-REN Morphodynamics Research group in Hungarian Space Caleidoscope 2023/24. It is related to the research on the elongated shape of Oumuamua.
Public lecture of Sándor Bozóki on monostable convex polyhedra
A public lecture has been presented within the framework of Celebration of Hungarian Science (“Magyar tudomány ünnepe”) by Sándor Bozóki. The lecture (in Hungarian) is available at the following link:
Proof of non-existence of mono-unstable polyhedra by means of inequalities (Monoinstabil poliéderek nemlétezésének igazolása egyenlőtlenségekkel)
Institutional Scientific Students’ Associations Conference 2023
At this year’s Institutional Scientific Students’ Associations Conference at the Budapest University of Technology and Economics, five presentations were related to Morphodynamics: Máté Szondi (1st Prize + Pro Progressio Special Prize), Gergő Almádi (1st Prize), Gergő Almádi and Eszter Ferencz (3rd Prize), Kinga Kocsis (Special award of the Department of Geometry and Morphology + Special award for presentation), Emese Sarolta Encz and Gergely Barta (Special award of the Department of Geometry and Morphology).
Continue Reading “Institutional Scientific Students’ Associations Conference 2023”
Public lecture of András Sipos on shape evolution in Nature
 A public lecture has been presented within the framework of Celebration of Hungarian Science (“Magyar tudomány ünnepe”) by András Sipos. The lecture (in Hungarian) is available at the following link:
A public lecture has been presented within the framework of Celebration of Hungarian Science (“Magyar tudomány ünnepe”) by András Sipos. The lecture (in Hungarian) is available at the following link:
Geometry and evolution of shapes in Nature (Geometria és alakfejlődés a természetben)
Applied Mathematics Day 2023
Two lectures will be presented at BME Applied Mathematics Day 2023, organized on 5th December, BME building Q, auditorium AF14.

A portrait of Gábor Domokos in MIT Technology Review
 A professional portrait of Gábor Domokos, leader of the HUN-REN-BME Morphodynamics Researh Group, has recently been published in MIT Technology Review by Elise Cutts.
A professional portrait of Gábor Domokos, leader of the HUN-REN-BME Morphodynamics Researh Group, has recently been published in MIT Technology Review by Elise Cutts.
Guest lecture: Rogério Martins
Why are we not able to see beyond three dimensions?
Rogério Martins, Universidade Nova de Lisboa
October 24, 2023
 Our guest lecturer gave a talk on problems of perception of higher dimensions in Nature.
Our guest lecturer gave a talk on problems of perception of higher dimensions in Nature.
New paper about the equilibria of tetrahedra
On Equilibria of Tetrahedra
Gergő Almádi, Robert J. MacG. Dawson, Gábor Domokos, and Krisztina Regős.
The paper focuses on the number of stable and unstable equilibria of tetrahedra with different mass distributions. The authors show that monostable tetrahedra exists with a suitably chosen distribution of density and, if a tetrahedron is monostable on one face, it can be made monostable on any other face with another distribution of density. Beyond tetrahedra, a construction for mono-monostatic pentahedra is also given in the paper.
Sándor Bozóki nominated full professor
 Sándor Bozóki, member of the HUN-REN Morphodynamics Research Group has been nominated full professor by the President of Republic on 5th September. Congratulations!
Sándor Bozóki, member of the HUN-REN Morphodynamics Research Group has been nominated full professor by the President of Republic on 5th September. Congratulations!
New paper on a 21-vertex mono-monostatic object with point masses
Conway’s Spiral and a Discrete Gömböc with 21 Point Masses
Gábor Domokos, Flórián Kovács.
Abstract: We show an explicit construction in three dimensions for a convex, mono-monostatic polyhedron (i.e., having exactly one stable and one unstable equilibrium) with 21 vertices and 21 faces. This polyhedron is a 0-skeleton, with equal masses located at each vertex. The above construction serves as an upper bound for the minimal number of faces and vertices of mono-monostatic 0-skeletons and complements the recently provided lower bound of 8 vertices. This is the first known construction of a mono-monostatic polyhedral solid. We also show that a similar construction for homogeneous distribution of mass cannot result in a mono-monostatic solid.
New paper on a possible evolution model of crack networks and other natural patterns
An Evolution Model for Polygonal Tessellations as Models for Crack Networks and Other Natural Patterns
Péter Bálint, Gábor Domokos, Krisztina Regős.
Abstract: We introduce and study a general framework for modeling the evolution of crack networks. The evolution steps are triggered by exponential clocks corresponding to local micro-events, and thus reflect the state of the pattern. In an appropriate simultaneous limit of pattern domain tending to infinity and time step tending to zero, a continuous time model, specifically a system of ODE is derived that describes the dynamics of averaged quantities. In comparison with the previous, discrete time model, studied recently by two of the present three authors, this approach has several advantages. In particular, the emergence of non-physical solutions characteristic to the discrete time model is ruled out in the relevant nonlinear version of the new model. We also comment on the possibilities of studying further types of pattern formation phenomena based on the introduced general framework.
Klaudia Nagy awarded as the student of the year
 Klaudia Nagy, member of the Morphodynamics Research Group, has been awarded as “Student of the Year” at the Faculty of Architecture of BME. Congratulations!
Klaudia Nagy, member of the Morphodynamics Research Group, has been awarded as “Student of the Year” at the Faculty of Architecture of BME. Congratulations!
Rocking stones in Tasmania
 ENSHRINE is a group of volunteer researchers, dedicated to preserve the natural heritage of Tasmania, Australia. Their studies on the behaviour of rocking stones, found in a large number in the area, were also based on some results of the MTA-BME Research Group for Morphodynamics (https://listthemountain.org/natural-features/rocking-stone).
ENSHRINE is a group of volunteer researchers, dedicated to preserve the natural heritage of Tasmania, Australia. Their studies on the behaviour of rocking stones, found in a large number in the area, were also based on some results of the MTA-BME Research Group for Morphodynamics (https://listthemountain.org/natural-features/rocking-stone).
Krisztina Regős in the “top 30 under 30” by Forbes.hu
Krisztina Regős, PhD student of the Morphodynamics Research Group was mentioned among the top 30 of most successful people under 30 in Hungary by Forbes.
Congratulations!
Guest lecture: Douglas J. Jerolmack
How things fall apart: The shape of failure across the solar system
Douglas J. Jerolmack, University of Pennsylvania
April 28, 2023
 Our guest lecturer, second time since 2019, gave a talk on various aspects of fragmentation in Nature.
Our guest lecturer, second time since 2019, gave a talk on various aspects of fragmentation in Nature.
New paper on the smallest mono-unstable convex polyhedron with point masses
The smallest mono-unstable convex polyhedron with point masses has 8 faces and 11 vertices
Dávid Papp, Krisztina Regős, Gábor Domokos, Sándor Bozóki
Abstract: In the study of monostatic polyhedra, initiated by John H. Conway in 1966, the main question is to construct such an object with the minimal number of faces and vertices. By distinguishing between various material distributions and stability types, this expands into a small family of related questions. While many upper and lower bounds on the necessary numbers of faces and vertices have been established, none of these questions has been so far resolved. Adapting an algorithm presented in Bozóki et al. (2022), here we offer the first complete answer to a question from this family: by using the toolbox of semidefinite optimization to efficiently generate the hundreds of thousands of infeasibility certificates, we provide the first-ever proof for the existence of a monostatic polyhedron with point masses, having minimal number (V=11) of vertices (Theorem 3) and a minimal number (F=8) of faces. We also show that V=11 is the smallest number of vertices that a mono-unstable polyhedron can have in all dimensions greater than 1.
The World’s largest Gömböc on display in Paris
The “Gömböc” is on permanent display since 17th April, 2023 at Pompidou Centre, Paris. The largest copy of Gömböc ever made of a single piece of material was introduced in the presence of one of its inventors, Gábor Domokos.
National Scientific Students’ Associations Conference 2023
Several presentations were held in the session for Mathematics, Physics and Geosciences of the 36th National Scientific Students’ Associations Conference in relation with the Morphodynamics Research Group. Gergő Almádi has been awarded by 3rd prize for his presentation entitled Inhomogén politópok mechanikai komplexitása – avagy van-e egy tetraédernek lelke?, under the supervision of Gábor Domokos and Krisztina Regős. Ágoston Szesztay (Iteratív módon csonkolt poliéderek statikai egyenúlyáról) and Máté Szondi (A kvantummechanikai állapottér egy felbontása által indukált geometria) got special prizes.
Continue Reading “National Scientific Students’ Associations Conference 2023”
New paper on polygonal tessellations in nanochemistry
Polygonal tessellations as predictive models of molecular monolayers
Krisztina Regős et al.
Abstract: Molecular self-assembly plays a very important role in various aspects of technology as well as in biological systems. Governed by covalent, hydrogen or van der Waals interactions–self-assembly of alike molecules results in a large variety of complex patterns even in two dimensions (2D). Prediction of pattern formation for 2D molecular networks is extremely important, though very challenging, and so far, relied on computationally involved approaches such as density functional theory, classical molecular dynamics, Monte Carlo, or machine learning. Such methods, however, do not guarantee that all possible patterns will be considered and often rely on intuition. Here, we introduce a much simpler, though rigorous, hierarchical geometric model founded on the mean-field theory of 2D polygonal tessellations to predict extended network patterns based on molecular-level information. Based on graph theory, this approach yields pattern classification and pattern prediction within well-defined ranges. When applied to existing experimental data, our model provides a different view of self-assembled molecular patterns, leading to interesting predictions on admissible patterns and potential additional phases. While developed for hydrogen-bonded systems, an extension to covalently bonded graphene-derived materials or 3D structures such as fullerenes is possible, significantly opening the range of potential future applications.
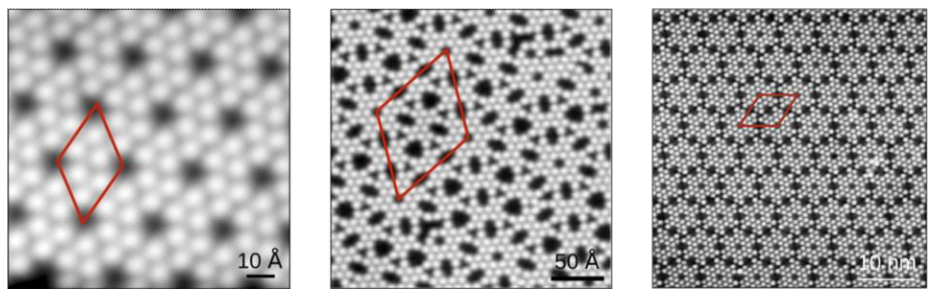
New paper on symmetry of mono-monostatic bodies
A characterization of the symmetry groups of mono-monostatic convex bodies
Gábor Domokos, Zsolt Lángi, Péter L. Várkonyi
Abstract: Answering a question of Conway and Guy (SIAM Rev. 11:78-82, 1969), Lángi (Bull. Lond. Math. Soc. 54: 501-516, 2022) proved the existence of a monostable polyhedron with n-fold rotational symmetry for any ?≥3, and arbitrarily close to a Euclidean ball. In this paper we strengthen this result by characterizing the possible symmetry groups of all mono-monostatic smooth convex bodies and convex polyhedra. Our result also answers a stronger version of the question of Conway and Guy, asked in the above paper of Lángi.
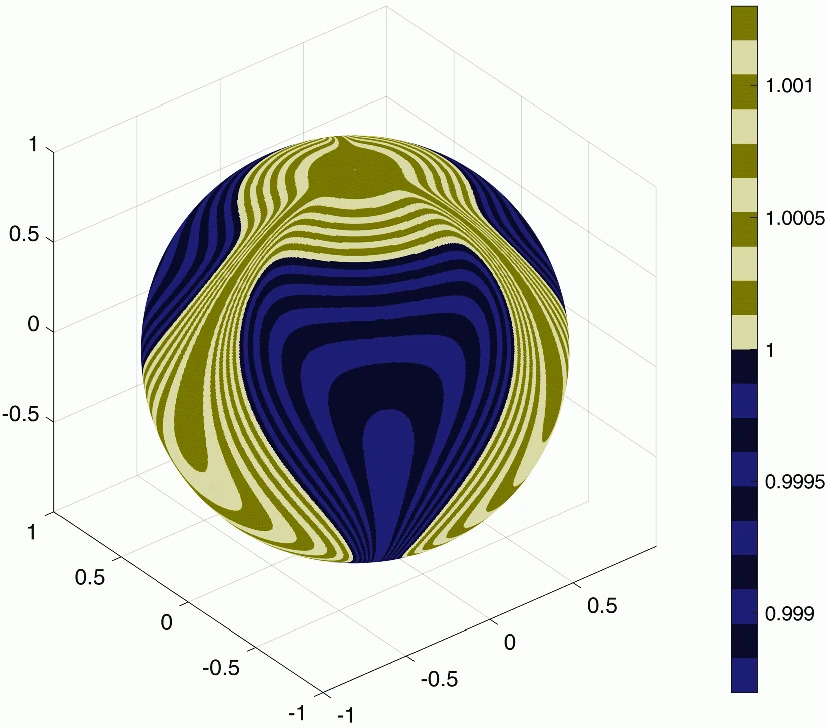
New paper on precariously balanced rocks
A New Insight into the Stability of Precariously Balanced Rocks
Balázs Ludmány, Ignacio Pérez-Rey, Gábor Domokos, Mauro Muñiz-Menéndez, Leandro R. Alejano, András Á. Sipos
Abstract: Recently it became increasingly evident that the statistical distributions of size and shape descriptors of sedimentary particles reveal crucial information on their evolution and may even carry the fingerprints of their provenance as fragments. However, to unlock this trove of information, measurement of traditional geophysical shape descriptors (mostly detectable on 2D projections) is not sufficient; fully spherical 3D imaging and mathematical algorithms suitable to extract new types of inherently 3D shape descriptors are necessary. Available 3D imaging technologies force users to choose either speed or full sphericity. Only partial morphological information can be extracted in the absence of the latter (e.g., LIDAR imaging). In the case of fully spherical imaging, speed was proved to be prohibitive for obtaining meaningful statistical samples, and inherently 3D shape descriptors were not extracted. Here we present a new method by complementing a commercial, portable 3D scanner with simple hardware to quickly obtain fully spherical 3D datasets from large collections of sedimentary particles. We also present software for the automated extraction of 3D shapes and automated measurement of inherently 3D-shape properties. This technique allows for examining large samples without the need for transportation or storage of the samples, and it may also facilitate the collaboration of geographically distant research groups. We validated our software on a large sample of pebbles by comparing previously hand-measured parameters with the results of automated shape analysis. We also tested our hardware and software tools on a large pebble sample in Kawakawa Bay, New Zealand.

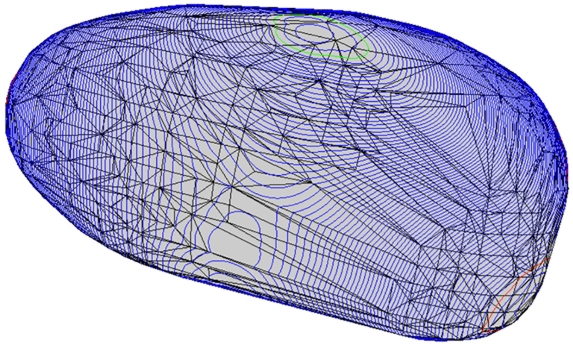
New paper on 3D pebble scanning
Fully spherical 3D datasets on sedimentary particles: Fast measurement and evaluation
Eszter Fehér, Balázs Havasi-Tóth, Balázs Ludmány
Abstract: Recently it became increasingly evident that the statistical distributions of size and shape descriptors of sedimentary particles reveal crucial information on their evolution and may even carry the fingerprints of their provenance as fragments. However, to unlock this trove of information, measurement of traditional geophysical shape descriptors (mostly detectable on 2D projections) is not sufficient; fully spherical 3D imaging and mathematical algorithms suitable to extract new types of inherently 3D shape descriptors are necessary. Available 3D imaging technologies force users to choose either speed or full sphericity. Only partial morphological information can be extracted in the absence of the latter (e.g., LIDAR imaging). In the case of fully spherical imaging, speed was proved to be prohibitive for obtaining meaningful statistical samples, and inherently 3D shape descriptors were not extracted. Here we present a new method by complementing a commercial, portable 3D scanner with simple hardware to quickly obtain fully spherical 3D datasets from large collections of sedimentary particles. We also present software for the automated extraction of 3D shapes and automated measurement of inherently 3D-shape properties. This technique allows for examining large samples without the need for transportation or storage of the samples, and it may also facilitate the collaboration of geographically distant research groups. We validated our software on a large sample of pebbles by comparing previously hand-measured parameters with the results of automated shape analysis. We also tested our hardware and software tools on a large pebble sample in Kawakawa Bay, New Zealand.
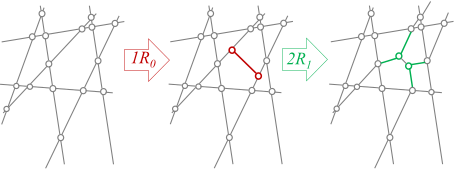
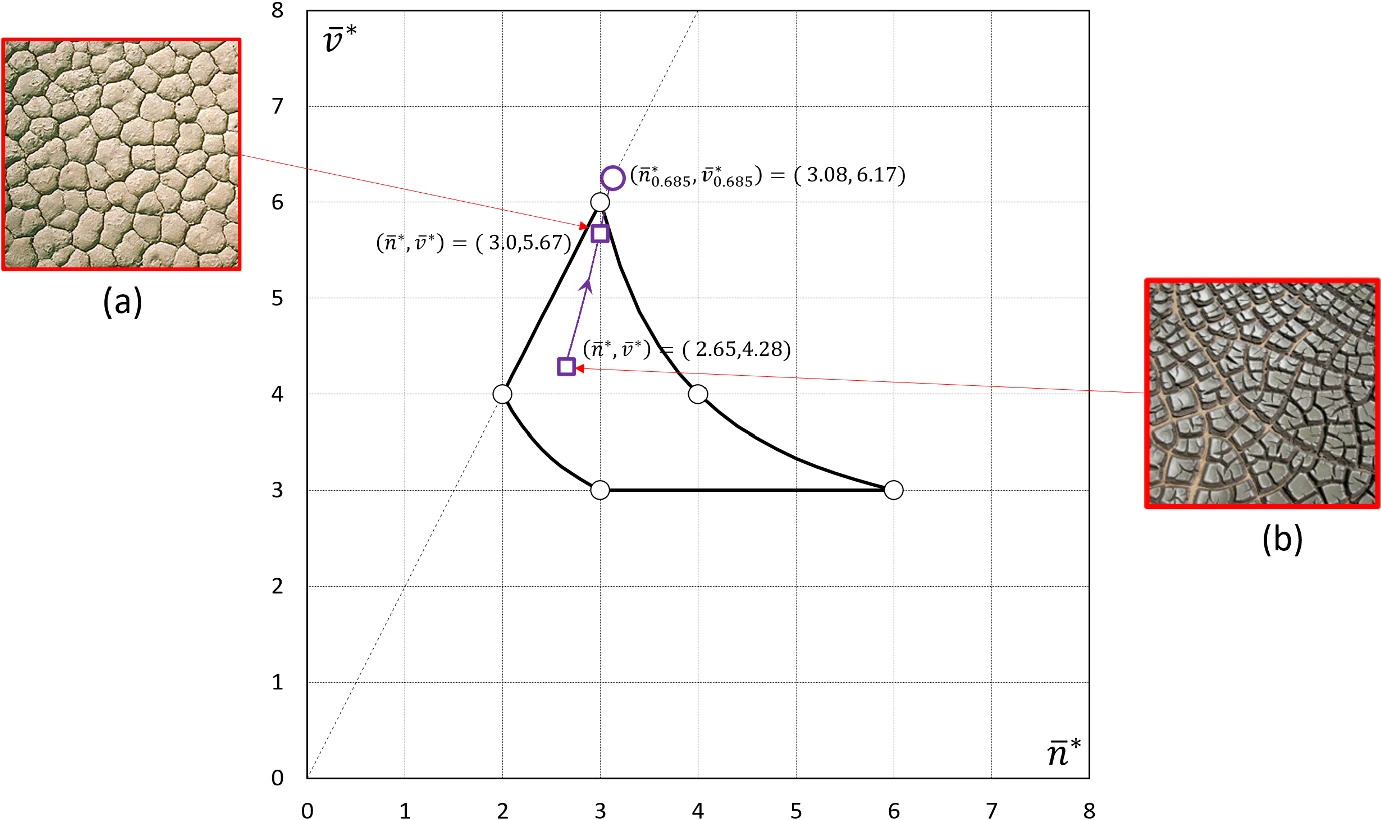
New paper on the evolution of fracture networks
A discrete time evolution model for fracture networks
Gábor Domokos, Krisztina Regős
Abstract: We examine geological crack patterns using the mean field theory of convex mosaics. We assign the pair (n̅∗, v̅∗) of average corner degrees to each crack pattern and we define two local, random evolutionary steps R0 and R1, corresponding to secondary fracture and rearrangement of cracks, respectively. Random sequences of these steps result in trajectories on the (n̅∗, v̅∗) plane. We prove the existence of limit points for several types of trajectories. Also, we prove that cell density ρ̅=n̅∗/v̅∗ increases monotonically under any admissible trajectory.
New paper on k-diametral point configurations
On k-diametral point configurations in Minkowski spaces
Károly Bezdek, Zsolt Lángi
Abstract: The structure of k-diametral point configurations in Minkowski d-space is shown to be closely related to the properties of k-antipodal point configurations in ℝd. In particular, the maximum size of k-diametral point configurations of Minkowski d-spaces is obtained for given k≥2 and d≥2 generalizing Petty’s results on equilateral sets in Minkowski spaces. Furthermore, bounds are derived for the maximum size of k-diametral point configurations in given Minkowski d-space (resp., Euclidean d-space). Some of these results have analogues for point sets, which are discussed as well. In the proofs convexity methods are combined with volumetric estimates and combinatorial properties of diameter graphs.
Interview with Gábor Domokos at perpal.hu
An interview with Gábor Domokos, one of the discoverers of Gömböc has recently been published at perpal.hu.
Beyond the history of Gömböc, the talk highlights the mutual impact the discovery and scientific thinking may have on each other.
Recognition of Zsolt Lángi at the Celebration of Hungarian Science
At the ceremony on 21 November, organized as part of the Celebration of Hungarian Science program series, Zsolt Lángi was also given a recognition by the Rector of University for having received the “Bolyai plakett” this year.
Congratulations!
Institutional Scientific Students’ Associations Conference 2022
At this year’s Institutional Scientific Students’ Associations Conference at the Budapest University of Technology and Economics, 4 presentations were related to Morphodynamics: Gergő Almádi (1st Prize + Pro Progressio Special Prize), Ágoston Szesztay (1st Prize), Klaudia Nagy (Csonka Pál Special Prize), Balázs Sárossi (2nd Prize).
Continue Reading “Institutional Scientific Students’ Associations Conference 2022”
Abrasion experiments at Centre de Recherches Pétrographiques et Géochimiques, Nancy
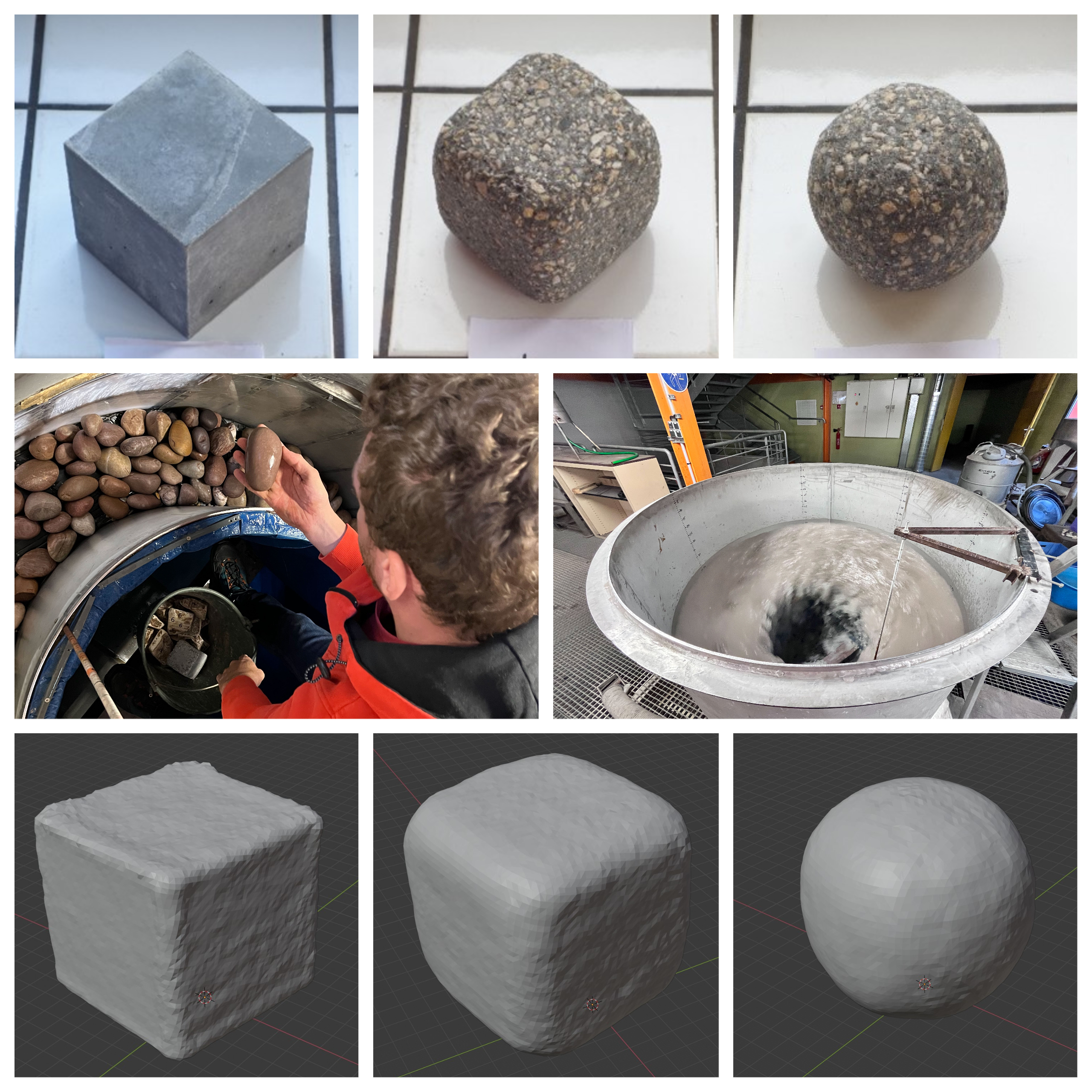 This October, Eszter Fehér and Balázs Havasi-Tóth visited Jérôme Lavé in the Centre de Recherches Pétrographiques et Géochimiques, Nancy to carry out abrasion experiments on concrete and sandstone cubes in a Flume. The concrete cubes were identified by RFID tags. During the experiments, the geometry of the abraded cubes was 3D scanned and their evolution was compared to theoretical predictions of abrasion models. It was also investigated how the movement of the pebbles depend on the pebble shape in the artificial river conditions of a Flume.
This October, Eszter Fehér and Balázs Havasi-Tóth visited Jérôme Lavé in the Centre de Recherches Pétrographiques et Géochimiques, Nancy to carry out abrasion experiments on concrete and sandstone cubes in a Flume. The concrete cubes were identified by RFID tags. During the experiments, the geometry of the abraded cubes was 3D scanned and their evolution was compared to theoretical predictions of abrasion models. It was also investigated how the movement of the pebbles depend on the pebble shape in the artificial river conditions of a Flume.
The concrete cubes were designed and created by Károly Péter Juhász, JKP Static. Here is a video of the concreting and the installation of RFID tags:
Applied Mathematics Without Borders
A series of lectures were presented at BME Applied Mathematics Day – Applied Mathematics Without Borders 2022, organized on 5-6 September, BME central building, 1st floor, “Díszterem” on the occasion of the 60th anniversary of Gábor Domokos.

Bolyai plakett for Zsolt Lángi
Zsolt Lángi, member of our research group, received the “Bolyai Plakett” award of the MTA in recognition of his three-year excellent work within the framework of the János Bolyai Research Scholarship.
Interview with Krisztina Regős at forbes.hu
An interview with Krisztina Regős, graduate student of the Faculty of Architecture of BME and her supervisor, Gábor Domokos has recently been published at forbes.hu.
The conversation reveals how Gömböc inspires young researchers, specifically how this discovery influenced Krisztina’s further research.
New paper on plane tilings
A two-vertex theorem for normal tilings
Gábor Domokos, Ákos G. Horváth, Krisztina Regős
Abstract: We regard a smooth, ?=2-dimensional manifold ℳ and its normal tiling M, the cells of which may have non-smooth or smooth vertices (at the latter, two edges meet at 180 degrees.) We denote the average number (per cell) of non-smooth vertices by ?¯⋆ and we prove that if M is periodic then ?¯⋆≥2. We show the same result for the monohedral case by an entirely different argument. Our theory also makes a closely related prediction for non-periodic tilings. In 3 dimensions we show a monohedral construction with ?¯⋆=0.

BME Applied Mathematics Day 2022.
Lecture at the Stuart Weitzman School of Design
Gábor Domokos will give a lecture “The invisible cube” at the University of Pennsylvania Stuart Weitzman School of Design on 16th February, at 6pm (Central European Time). The lecture gives insight into the work of the Morphology and Applied Geometry Department and the Morphodynamics research group. Follow the lecture online: zoom link.

Institutional Scientific Students’ Associations Conference 2021
The Morphodynamics Group had a session titled ‘Geometry’ in the 2021 TDK Conference. Five students participated in the session and received numerous awards. Congratulations!
Krisztina Regős (1st Prize + Rector’s Award), Anna Viczián (1st Prize), Ágoston Szesztay (3rd Prize + Csonka Pál Special Prize), Klaudia Nagy (Department’s Special Prize), Máté Szondi (Metszet Journal Special Prize).
Continue Reading “Institutional Scientific Students’ Associations Conference 2021”
New paper on Mono-unstable polyhedra
Mono-unstable polyhedra with point masses have at least 8 vertices
Sándor Bozóki, Gábor Domokos, Flórián Kovács, Krisztina Regős
Abstract: The monostatic property of convex polyhedra (i.e., the property of having just one stable or unstable static equilibrium point) has been in the focus of research ever since Conway and Guy (1969) published the proof of the existence of the first such object, followed by the constructions of Bezdek (2011) and Reshetov (2014). These examples establish
as the respective upper bounds for the minimal number of faces and vertices for a homogeneous mono-stable polyhedron. By proving that no mono-stable homogeneous tetrahedron existed, Conway and Guy (1969) established for the same problem the lower bounds for the number of faces and vertices as
and the same lower bounds were also established for the mono-unstable case (Domokos et al., 2020b). It is also clear that the
bounds also apply for convex, homogeneous point sets with unit masses at each point (also called polyhedral 0-skeletons) and they are also valid for mono-monostatic polyhedra with exactly one stable and one unstable equilibrium point (both homogeneous and 0-skeletons). In this paper we draw on an unexpected source to extend the knowledge on mono-monostatic solids: we present an algorithm by which we improve the lower bound to
vertices on mono-unstable 0-skeletons. The problem is transformed into the (un)solvability of systems of polynomial inequalities, which is shown by convex optimization. Our algorithm appears to be less well suited to compute the lower bounds for mono-stability. We point out these difficulties in connection with the work of Dawson, Finbow and Mak (Dawson, 1985, Dawson et al., 1998, Dawson and Finbow, 2001) who explored the monostatic property of simplices in higher dimensions.
New paper on Curvature flows
Curvature flows, scaling laws and the geometry of attrition under impacts
Gergő Pál, Gábor Domokos & Ferenc Kun
Abstract: Impact induced attrition processes are, beyond being essential models of industrial ore processing, broadly regarded as the key to decipher the provenance of sedimentary particles. Here we establish the first link between microscopic, particle-based models and the mean field theory for these processes. Based on realistic computer simulations of particle-wall collision sequences we first identify the well-known damage and fragmentation energy phases, then we show that the former is split into the abrasion phase with infinite sample lifetime (analogous to Sternberg’s Law) at finite asymptotic mass and the cleavage phase with finite sample lifetime, decreasing as a power law of the impact velocity (analogous to Basquin’s Law). This splitting establishes the link between mean field models (curvature-driven partial differential equations) and particle-based models: only in the abrasion phase does shape evolution emerging in the latter reproduce with startling accuracy the spatio-temporal patterns (two geometric phases) predicted by the former.

Successful PhD defense
Congratulations to Sára Lévay, who successfully defended her PhD thesis: Self-organizing processes in granular materials (supervisor: János Török).

Another paper in Aequationes Mathematicae
An analogue of a theorem of Steinitz for ball polyhedra in R-3
Sami Mezal Almohammad, Zsolt Lángi, Márton Naszódi
Abstract: Steinitz’s theorem states that a graph G is the edge-graph of a 3-dimensional convex polyhedron if and only if, G is simple, plane and 3-connected. We prove an analogue of this theorem for ball polyhedra, that is, for intersections of finitely many unit balls in ℝ3.
Scanning pebbles in Siena
After our short visit and consultation in Sienna, Italy, before the global pandemic breakout, our colleagues finally had the opportunity to construct their own scanning equipment. We are looking forward to see their scanning results and experiences with the newly built devices.


Gomboc software in sailing
The Gomboc simulation software – whose name was chosen after the Gömböc of Gábor Domokos and Péter Várkonyi – played a crucial role in the the 36th America’s cup.
Read more (in Hungarian): A MAGYAR GÖMBÖCRŐL ELNEVEZETT GOMBOC SZOFTVERREL DIADALMASKODOTT A GYŐZTES CSAPAT A VILÁG LEGELITEBB VITORLÁSVERSENYÉN
An article about the Gomboc software in the 35th America’s cup (in English): Gomboc: A design high-flier for ETNZ
Science Magazine top 10
Science Magazine included the paper “Plato’s cube and the natural geometry of fragmentation” in the top 10 non-covid science news stories of 2020.
List of the top 10 science news stories of 2020:
Top news stories of 2020
Podcast episode:
Breakthrough of the Year, top online news, and science book highlights (9:36 – 12:40)
Institutional Scientific Students’ Associations Conference 2020
At this year’s Institutional Scientific Students’ Associations Conference at the Budapest University of Technology and Economics, 2 presentations were related to Morphodynamics: Ágoston Szesztay (1st Prize + Pro Progressio Special Prize), Klaudia Nagy (Csonka Pál Special Prize).
Continue Reading “Institutional Scientific Students’ Associations Conference 2020”
Quanta Magazine article
An outstanding article from Joshua Sokol Scientists Uncover the Universal Geometry of Geology about the paper “Plato’s cube and the natural geometry of fragmentation” in Quanta Magazine.
Krisztina Regős – Famelab 2020
Congratulations to Krisztina Regős, who won the audience favorite prize at Famelab 2020!
Article of the competiton by mta.hu
Article on bme.hu

photo: mta.hu / Tamás Szigeti
You can watch her talk here:
New paper on fragmentation
Plato’s cube and the natural geometry of fragmentation
G. Domokos, D.J. Jerolmack, F. Kun, J. Török
arxiv:1912.04628
Abstract: Plato envisioned Earth’s building blocks as cubes, a shape rarely found in nature. The solar system is littered, however, with distorted polyhedra—shards of rock and ice produced by ubiquitous fragmentation. We apply the theory of convex mosaics to show that the average geometry of natural two-dimensional (2D) fragments, from mud cracks to Earth’s tectonic plates, has two attractors: “Platonic” quadrangles and “Voronoi” hexagons. In three dimensions (3D), the Platonic attractor is dominant: Remarkably, the average shape of natural rock fragments is cuboid. When viewed through the lens of convex mosaics, natural fragments are indeed geometric shadows of Plato’s forms. Simulations show that generic binary breakup drives all mosaics toward the Platonic attractor, explaining the ubiquity of cuboid averages. Deviations from binary fracture produce more exotic patterns that are genetically linked to the formative stress field. We compute the universal pattern generator establishing this link, for 2D and 3D fragmentation.
The article was followed by increased media attention. Here is a list of the international and national mentions of the MTA-BME Morphodynamics Research Group: Media mentions.
New paper on Balancing polyhedra
Balancing polyhedra
G. Domokos, F. Kovács, Z. Lángi, K. Regős and P.T. Varga, Balancing polyhedra, Ars Math. Contemp., accepted, arXiv:1810.05382 [math.MG]
Abstract: We define the mechanical complexity C(P) of a convex polyhedron P, interpreted as a homogeneous solid, as the difference between the total number of its faces, edges and vertices and the number of its static equilibria, and the mechanical complexity C(S,U) of primary equilibrium classes (S,U)E with S stable and U unstable equilibria as the infimum of the mechanical complexity of all polyhedra in that class. We prove that the mechanical complexity of a class (S,U)E with S,U>1 is the minimum of 2(f+v−S−U) over all polyhedral pairs (f,v), where a pair of integers is called a polyhedral pair if there is a convex polyhedron with f faces and v vertices. In particular, we prove that the mechanical complexity of a class (S,U)E is zero if, and only if there exists a convex polyhedron with S faces and U vertices. We also give asymptotically sharp bounds for the mechanical complexity of the monostatic classes (1,U)E and (S,1)E, and offer a complexity-dependent prize for the complexity of the Gömböc-class (1,1)E.
KOMAL article on convex polyhedra
Article appeared in the High School Mathematics and Physics Journal (KOMAL) with the title: Equilibria of convex polyhedra (in Hungarian).
Authors: Gábor Domokos, Flórián Kovács, Zsolt Lángi, Krisztina Regős, Péter Tamás Varga
Zsolt Lángi ÚNKP success stories
Congratulations to Zsolt Lángi, who is listed in the New National Excellence Program (ÚNKP) success stories!
Details in Hungarian: ÚNKP success stories.
Krisztina Regős is a finalist in FameLab 2020
Krisztina Regős is a finalist in the FameLab Hungary 2020. The national finals will be in the Fall of 2020.
Interview with Gábor Domokos
Gábor Domokos recently received the precious Gábor Dénes award. Interview at bme.hu (in Hungarian).
Gábor Dénes Award 2019
Ice eggs and ooids
Interesting natural phenomenon explained on index by our research group.

Figure: Ooid sand from The Bahamas.
Guest lecture: Bernd Krauskopf
Excitability and feedback: to pulse or not to pulse?
Bernd Krauskopf (University of Auckland)
joint work with Soizic Terrien and Neil Broderick (University of
Auckland), Anirudh Pammi and Sylvain Barbay (C2N, Paris-Saclay)
18 November, 2019
Department of Physics, 4pm
Abstract: Excitability is a very common phenomenon in the dynamics of many natural and engineered systems; examples are neurons, certain chemical reactions and laser systems. Being at equilibrium, an excitable system reacts to a sufficiently large perturbation by suddenly releasing a pulse of stored energy. Then the system needs some time to recover its level of stored energy. When excitable systems are coupling to themselves or to each other, they receive feedback with a delay time that is considerably larger than the pulse length. This may lead to very interesting pulsing dynamics. We demonstrate this here with an excitable micropillar laser with a feedback loop, or external cavity, generated by a regular mirror, which has been shown experimentally to be able to sustain trains of optical pulses. These can be triggered largely independently by optical perturbations injected into the laser, and they are then sustained simultaneously via feedback from the external cavity. A bifurcation analysis of a rate-equation model shows that the system has a number of periodic solutions with different numbers of equally spaced pulses as its only attractors. Hence, although coexisting pulse trains can seem independent on the timescale of the experiment, they correspond to very long transient dynamics. We determine the switching dynamics by studying the associated basins of attraction, which demonstrates that timing is everything when it comes to triggering or erasing pulse trains.

Guest lecture: Alain Goriely
On the shape of gravitating planets
Alain Goriely, University of Oxford
October 22, 2019
Abstract: A classic problem of elasticity is to determine the possible equilibria of a planet modelled as a homogeneous compressible spherical elastic body subject to its own gravitational field. In the absence of gravity the initial radius is given and the density is constant. With gravity and for small planets, the elastic deformations are small enough so that the spherical equilibria can be readily obtained by using the theory of linear elasticity. For larger or denser planets, large deformations are possible and surprising behaviours emerge as will be revealed during this talk.

The geometry of turbulence, Applied Mathematics Day 2019
Székelyhidi László (Universität Leipzig):
Rigid surfaces from Euclid to Nash and how to bend them.
October 16, 2019
16.30 BME K.150
“A compact surface is called rigid if the only length-preserving transformations of it are congruencies of the ambient space – in simple terms rigid surfaces are unbendable. Rigidity is a topic which is already found in Euclid’s Elements. Leonhard Euler conjectured in 1766 that every smooth compact surface is rigid. The young Augustin Cauchy found a proof in 1813 for convex polyhedra, but it took another 100 years until a proof for smooth convex surfaces appeared. Whilst it seems clear that convexity, and more generally curvature plays an important role for rigidity, it came as a shock to the world of geometry when John Nash showed in 1954 that every surface can be bent in an essentially arbitrary, albeit non-convex manner. The proof of Nash involves a highly intricate fractal-like construction, that has in recent years found applications in many different branches of applied mathematics, such as the theory of solid-solid phase transitions and hydrodynamical turbulence. The talk will provide an overview of this fascinating subject and present the most recent developments.”


(Source: http://hevea-project.fr/)
Uriel Frisch (CNRS, France):
Leonardo da Vinci, Tódor Kármán and many more: the decay of turbulence.
October 16, 2019
17.30 BME K.150
“Leonardo had a strong interest in mathematics (at the time, mostly geometry and simple algebraic equations). In the early part of his life, spent in Florence, Leonardo became interested in chaotic hydrodynamics (called by him, for the first time “turbulence”), a topic which will persist throughout his life. Examining the “turbulences” (eddies) in the river Arno he found in the late 1470 that the amplitude of the turbulence was decreasing very slowly in time, until it would come to rest (within the surrounding river). This topic would remain dormant for close to 5 centuries, until in 1938 Todor (Theodore) Karman, triggered by Geoffrey Taylor, established that the amplitude of the turbulence should decrease very slowly, indeed like an inverse power of the time elapsed. Three years later, Andrei Kolmogorov found an algebraic mistake in Karman’s calculation; Kolmogorov himself found another inverse power (5/7) of the time elapsed. This, likewise was wrong. In the talk we will present developments in a historical context and connect to recent progress on this topic. Very recently, we found that the law of decay of the amplitude need not be exactly an inverse of the time elapsed. Furthermore, more exotic laws of decay were obtained for “weak” (distributional) solutions using a Nash-like construction.”


BME- NEWS: „I started research with the support of an excellent group of professors”
Talk at the DDG2 conference
Mechanical complexity of complex polyhedra
Flórián Kovács, MTA-BME Morphodynamics Research Group
July 10, 2019
Discrete Geometry Days 2, Budapest
Abstract: Let P be a convex polyhedron with f faces, e edges and v vertices, and assume it is realized as a homogeneous solid having S stable, H saddle-type and U unstable equilibrium configurations (i.e., standing on a face, edge or vertex over a horizontal surface). Let mechanical complexity C(P) of P be defined as the difference between the sums f + e + v and S + H + U and define mechanical complexity C(S,U) of primary equilibrium classes (S,U)^E as the minimum of mechanical complexities of all polyhedra having S stable and U unstable equilibria. In this talk we show that mechanical complexity in any non-monostatic (i.e., S > 1 and U > 1) primary equilibrium class (S,U)^E equals the minimum of 2(f+v−S−U) over all polyhedra in the same class having simultaneously f faces and v vertices; thus, mechanical complexity of a class (S,U)^E is zero if and only if there exists P such that f = S and v = U. We also give both upper and lower bounds for C(S,U) in the cases S = 1 ≠ U and U = 1 ≠ S (a problem related to a question of Conway and Guy in 1969 asking about the minimal number of faces of convex polyhedra with only one stable equilibrium point), and we offer a complexity-dependent prize for C(1,1). Joint work with Gábor Domokos, Zsolt Lángi, Krisztina Regős and Péter T. Varga.
Guest lecturer: Boris A. Khesin
Integrability of pentagram maps and KdV hierarchies
Boris A. Khesin, University of Toronto
June 27, 2019
Abstract: We describe pentagram maps on polygons in any dimension, which extend R.Schwartz’s definition of the 2D pentagram map. Many of those maps turn out to be discrete integrable dynamical systems, while the corresponding continuous limits of such maps coincide with equations of the KdV hierarchy, generalizing the Boussinesq equation in 2D. We discuss their geometry, Lax forms, and interrelations between several recent pentagram generalizations.

National Scientific Conference Presentation Award 2019
Congratulations for Krisztina Regős on winning one of the four Presentation Awards on the National Scientific Student’s Conference 2019 with her presentation titled ‘Morphological analysis of fractured rock surfaces‘.
Applied Mathematics Day 2019.
Denis Weaire FRS (Trinity College, Dublin)
On growth and form (of bubbles)
29th April, 2019. 16:00
BME K150
“Bubbles and foams have always attracted the interest of artists, poets and philosophers (as well as small children). Physicists are similarly drawn to the subject, but with more precise aims – to understand the detailed structure of crowded bubbles and their competing growth, as well as their mechanical properties. Here we will concentrate mainly on structure, recalling the contributions of Plateau and Kelvin in the 19th century and their echoes down to the present time.”


Contributors: Douglas J. Jerolmack and Ferenc Kun
Douglas J. Jerolmack and Ferenc Kun attended our seminar on 13th April, 2019.


Insulin capsules inspired by the Gomboc
National Scientific Student’s Conference 2019
Results of the National Scientific Student’s Conference 2019:
Krisztina Regős Special prize
Péter Varga 2nd Prize
Congratulations to both of them!
Guest: Michel Potier-Ferry
Our guest Michel Potier-Ferry from University of Lorraine, France was a Reviewer and Member of the Doctoral committee at the PhD defense of Eszter Fehér.

PhD defense
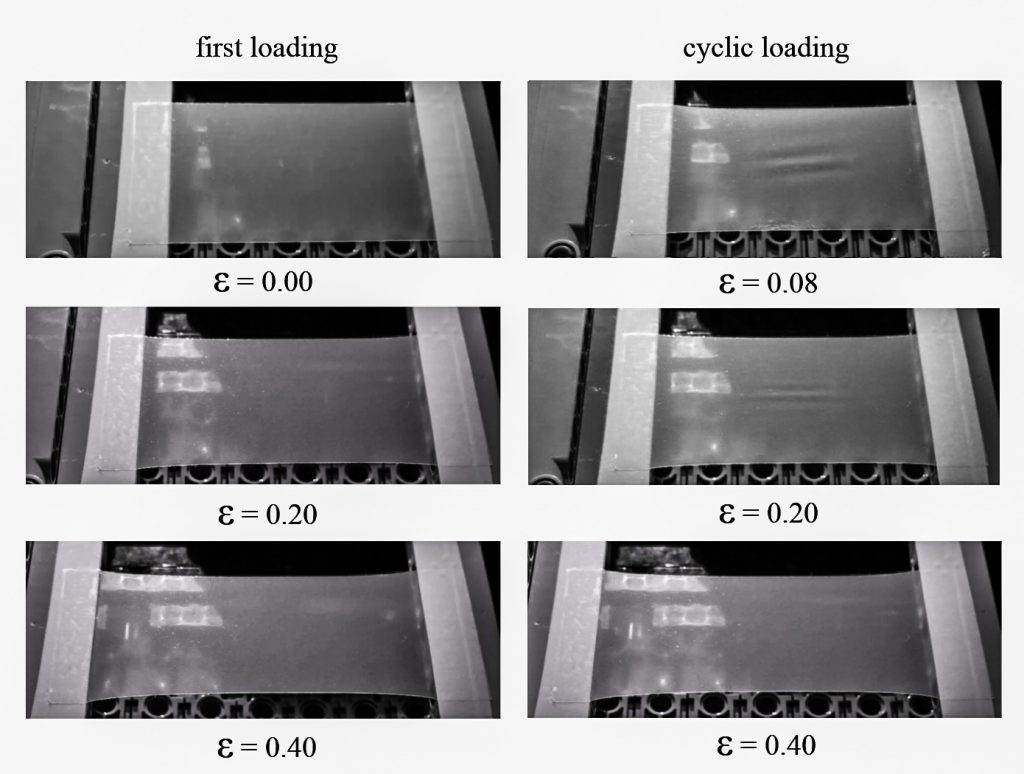
Our member Eszter Fehér successfully defended her PhD thesis Wrinkling behavior of highly stretched thin films.
Brief summary: In the literature of the wrinkling of ultrathin films, theoretical models were often used to investigate phenomena that fall outside the range of validity of their assumptions. Contradictory results were published regarding the wrinkling of axially stretched, clamped, rectangular films. Based on the Föppl-von Kármán plate theory (FvK) wrin- kles appear on the surface and their amplitude scales with the macroscopic strain. A finite membrane strain extension of the FvK theory (eFvK) predicted that only a bounded region of the macroscopic strain and aspect ratio parameters exhibit wrinkling in the problem.
The first goal of the work is to verify these predictions experimentally. Secondly, the eFvK model was extended into directions, that are beneficial in the modeling of real-life structures. The effect of material and geometrical properties and nonlinearities on wrinkling was also examined in detail.
Orthotropy was incorporated into the model and its effect was investigated. By comparing the results of the orthotropic model and experiments carried out on pre- stressed polyurethane films, the predictions of the eFvK model were experimentally verified. Motivated by further experimental observations, a pseudoelastic model based on the Mullins effect was also proposed and it was shown, that it can explain the intriguing phenomenon arisen during the prestressing of polyurethane films.
The eFvK model was extended to curved surfaces, and it was shown, that the intrinsic curvature can reduce wrinkling. Finally, we carried out an investigation of the parameter space by letting the thickness of the film arbitrary small. We introduced the concept of disturbed zones near the clamps which gave a physical explanation of the aspect ratio dependency of the wrinkling in the model problem.In the literature of the wrinkling of ultrathin films, theoretical models were often used to investigate phenomena that fall outside the range of validity of their assumptions. Contradictory results were published regarding the wrinkling of axially stretched, clamped, rectangular films. Based on the Föppl-von Kármán plate theory (FvK) wrin- kles appear on the surface and their amplitude scales with the macroscopic strain. A finite membrane strain extension of the FvK theory (eFvK) predicted that only a bounded region of the macroscopic strain and aspect ratio parameters exhibit wrinkling in the problem.
The first goal of the work is to verify these predictions experimentally. Secondly, the eFvK model was extended into directions, that are beneficial in the modeling of real-life structures. The effect of material and geometrical properties and nonlineari- ties on wrinkling was also examined in detail.
Orthotropy was incorporated into the model and its effect was investigated. By comparing the results of the orthotropic model and experiments carried out on prestressed polyurethane films, the predictions of the eFvK model were experimentally verified. Motivated by further experimental observations, a pseudoelastic model based on the Mullins effect was also proposed and it was shown, that it can explain the intriguing phenomenon arisen during the prestressing of polyurethane films.
The eFvK model was extended to curved surfaces, and it was shown, that the intrinsic curvature can reduce wrinkling. Finally, we carried out an investigation of the parameter space by letting the thickness of the film arbitrary small. We introduced the concept of disturbed zones near the clamps which gave a physical explanation of the aspect ratio dependency of the wrinkling in the model problem.
Institutional Scientific Students’ Associations Conference 2018
At this year’s Institutional Scientific Students’ Associations Conference at the Budapest University of Technology and Economics, 3 presentations were related to Morphodynamics: Krisztina Regős (1st Prize + Pro Progressio Special Prize), Dániel Csallóközi (2nd Prize + Csonka Pál Special Prize), Péter Tamás Varga (3rd Prize).
Continue Reading “Institutional Scientific Students’ Associations Conference 2018”
Guest lecturer: Sir Michael Berry
Nature’s optics and our understanding of light
Sir Michael Berry
University of Bristol
December 12th, 2018 at 4 pm
BME, K134
Abstract: Optical phenomena visible to everyone abundantly illustrate important ideas in science and mathematics. The phenomena considered include rainbows, sparkling reflections on water, green flashes, earthlight on the moon, glories, daylight, crystals, and the squint moon. The concepts include refraction, wave interference, numerical experiments, asymptotics, Regge poles, polarization singularities, conical intersections, and visual illusions.

Guest lecturer: Bernd Krauskopf
Computing global invariant manifolds as a sequence of geodesic level sets
Bernd Krauskopf, University of Auckland
joint work with
Hinke Osinga, University of Auckland
May 16, 2018
Abstract: Steady-states and periodic orbits of saddle type of dynamical systems defined by ordinary differential equations come with stable and unstable manifolds. These invariant, global objects play a crucial role in organising the dynamics, for example, as basin boundaries and in the creation of chaotic attractors. We consider here global invariant manifolds of dimension two in three-dimensional systems. These are implicitely defined surfaces that can generally only be found by numerical means.
We present arguably the most geometric approach to finding and visualising these surfaces: we grow a manifold step by step from the steady-state of periodic orbit by computing a sequence of rings or bands at suitable geodesic distances. Our method is illustrated with several examples, including the Lorenz system.




